A lot of hedge fund managers seem to believe that the Fed and other central banks that have been using unconventional monetary policy, specifically “quantitative easing” (see note 1), are dooming us to much higher inflation in future. Some believe the very large increase in central bank balance sheets will cause hyperinflation, economic ruin and the collapse of western civilisation. I’m not exaggerating, this is a constant theme of some of the hedge fund blogs and newsletters. At a recent MFin finance alumni event two hedgies argued that inflation was inevitable, one privately, the other on the panel. They clearly believe it, and with some seriousness. Yet so far, after four years during which quantitative easing has been followed by lower inflation and until recently record low government bond yields, they have been completely wrong.
I note that these hedgies are typically not global macro types who are experts on the macroeconomy. Those investors vary more in their views. The hedgies convinced that inflation is coming are more often than not the stock pickers or value investors. They are, if you like, micro-players, who don’t attempt to time the whole market. The whole point of a hedge fund is that it hedges out market risk, so inflation isn’t that important except to the extent that it affects different parts of the economy (and so different stocks) in a varying way. And that’s not normally part of the hedgie inflation worry, they usually are concerned about the whole macroeconomy and the ruination of finance as we know it.
Where does this view come from? I have a hunch that it’s based on a little knowledge of inflation economics that these guys (they are usually guys) picked up either at business school or along the way from other financial experts who didn’t do much economics. I think that in short, they are haunted by a memory of the quantity theory of money.
To explain what is causing the hedgies anxiety, this chart shows the huge increase in the balance sheets of the US and UK central banks. Both the Fed and Bank of England have bought large amounts of financial assets, such as government bonds and mortgage backed securities, funded by the creation of new reserves, that is new money owned by the commercial banks from whom the assets were bought.
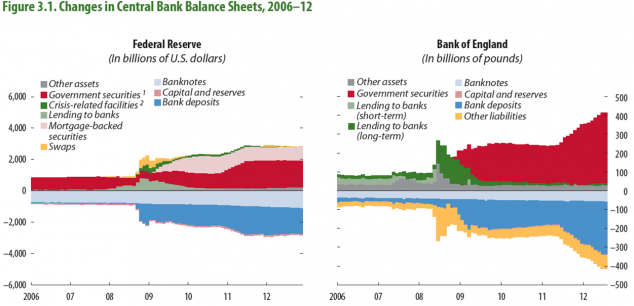
(The Cleveland Fed has a more detailed analysis of what they call “credit easing” here). There is no historical precedent for this expansion of central bank activities, so it’s quite reasonable to be concerned about it and how it might be withdrawn safely when economic recovery has taken hold. But does a large expansion of these commercial bank deposits necessarily imply inflation?
The quantity theory of money
The quantity theory of money goes back to the classical economists David Hume and John Stuart Mill in the nineteenth century. But the basic idea is far older than that (Wikipedia cites Copernicus, writing in the early sixteenth century). It says that the value of money, or equivalently the price of things which money buys (the overall price level), depends on how much money there is. So if there is an increase in the amount of money in circulation, with nothing else changing, then the price level will rise, meaning there is inflation.
The most famous historic example of this is usually taken to be the rise of inflation in Spain and then much of the rest of Europe in the sixteenth century, following the huge increase in the amount of gold and silver entering the economy from the “New World” (central and southern America). These precious metals were used as money, in coin form, so a large, exogenous increase in the amount led, as the quantity theory would predict, to inflation. There are other, competing explanations for the inflation, but the general idea seems plausible.
The more modern expression of the quantity theory is an equation that is simple but gives us enough to explore why this persuasive account of inflation with physical, commodity money, doesn’t work very well with modern, fiat (government backed and bank created) money.
MV = PT……(1)
Equation (1) is actually an identity, it is true by definition.
M is the stock of money in the economy.
V is the velocity of circulation of money.
P is the average price level.
T (it’s sometimes written as Y) is the total real volume of output in the economy.
So PT is the total nominal value of economic output – the real quantity multiplied by the price at which output is sold.
MV is the total value of monetary transactions used to buy the economic output. It must be true that this equals the value of what is produced and sold.
V is the puzzling thing here. It is easiest to think of it as a stock-flow ratio, by rearranging equation (1) like this:
V = PT/M……(2)
Equation (2) says that V is the ratio of the total volume of nominal output (an annual flow) divided by the stock of money defined at a point in time (say the end of the year, or perhaps the average during the whole of the year).
Equations (1) and (2) don’t tell us anything other than definitions. To turn them into a testable (in principle) theory, we need to make some assumptions.
Turning an identity into a hypothesis
It is a long established view among many economists that the real economy is determined by real things such as the amount of labour supply, and the amount and productivity of capital. If this is true, then T is given by these real factors, independently of the level of inflation or anything else monetary. This is known as the Classical Dichotomy. Keynes and other mid-twentieth century economists argued that this dichotomy was false and that monetary condtions can affect the functioning of the real economy. But many economists, especially non-Keynesian ones, still believe it to be broadly true.
If we assume that T is fixed and we make the further assumption that the stock-flow ratio V is also fixed (more on that in a moment) then we are left with the logical conclusion that changes in M, the money supply, are directly reflected in changes in P, the average price level. Increases in money supply cause inflation, if our two assumptions are correct. It would also be true that changes in P could cause changes in M, but it’s harder to think of how that could about and the quantity theory usually assumes the money supply is either exogenous (e.g. it depends on the amount of gold and silver mined) or under the control of a government or central bank.
When money is a simple commodity like gold or silver, it’s not difficult to see how a sudden exogenous rise in money will probably lead to inflation, though perhaps after a lag. Initially somebody has extra gold (perhaps they dug it up in a new gold mine) and this is accepted by shop keepers and others as having just the same value as the existing gold. But as the gold circulates in the economy, and with the same production of actual, real resources, then eventually the value of gold must fall somewhat as people realise it is not as scarce as it used to be. If gold in circulation rises by say 10% and real output is the same, then the eventual equilibrium is that the price of gold falls by 10%, which is equivalent to general inflation of 10% (the purchasing value of gold has fallen by 10%).
This story, perhaps we should call it a parable, can be extended to money in the form of bank notes printed by a government-backed central bank, which is the system prevailing in most countries following the end of gold and silver coins in circulation. Imagine there is a fixed amount of physical notes in circulation and a certain level of real output. There is a certain purchasing power for each note and zero inflation at first.
If the government tries to print more notes, without there being any change in the level of national output, then soon people will spot that the notes are more plentiful and so less valuable and there will be inflation. If there is a very large increase in the amount of printed notes then people will be very reluctant to hold them and will spend them as soon as possible. With everyone in the economy acting (rationally) this way, the value of the notes will plummet and we have hyperinflation.
The hedgies, seeing the very large increase in the volume of money created by central banks in the policy of quantitative easing, draw the conclusion that inflation simply must follow. But that new money is not actually printed, indeed virtually none of it is in physical form. It’s in the form of reserves created to buy securities such as government bonds from banks. In a modern, credit-based economy, the simple argument from the quantity theory doesn’t work so well, indeed it’s not even clear how to apply it. To quote an excellent t-shirt slogan, I think you’ll find it’s a bit more complicated than that. First of all, we’re no longer at all sure what is “money”.
What is money?
In our examples above it was absolutely clear what money was – a certain quantity of gold coins or printed bank notes. But in today’s economy the great majority of what is money is bank deposits and other financial assets. This creates a serious problem of what is the quantity of money? Central banks have multiple definitions of “narrow” and “broad” money, depending on whether focus on just notes and coins or include checking (current) deposits, savings deposits, certificates of deposits, money market funds and even treasury bills. Anything that can fulfill the functions of money (means of payment, store of value) can be money.
The chart below shows the annual percentage changes in six different definitions of money. The last two are weighted categories of money using the divisia approach, an arguably superior measure to the usual simple average index, associated with the Center for Financial Stability.
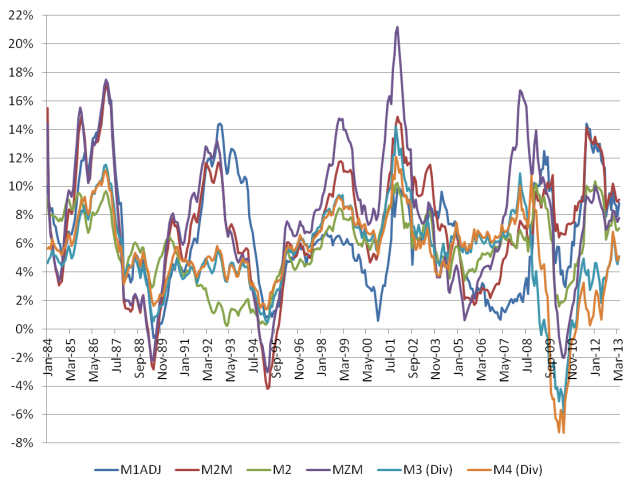
M1 – notes and coins in circulation plus demand and other checkable bank accounts
M2 – M1 plus savings and small time deposit accounts (up to $100,000)
M2M – M2 but weighted according to estimates of the monetary services yielded to users
M3 – M2 plus large time deposit accounts
MZM – (zero maturity assets) – M1 plus savings deposits and money market funds
M4 – (not a Fed measure) – M3 plus money market funds, commercial paper and T-bills
Depending on the measure of money supply you choose, the annual change can vary enormously and not in a predictable way. Central banks that tried to target the money supply during the period of “monetarism” in the 1980s eventually gave up because of the problem of defining and measuring that which they were trying to control. Bank of England chief economist Charles Goodhart gave his name to a “law” that states that “ny observed statistical regularity will tend to collapse once pressure is placed upon it for control purposes.” In other words, even if central banks could define money, attempts to control it for the goal of controlling inflation would fail.
A second problem is the assumption that T, real output, is given independently of monetary conditions. One of the lessons of the credit crisis and its aftermath, especially in the Eurozone, is that the real economy can malfunction badly if the financial system is damaged. Real output is not, at least in the current conditions, solely determined by real factors. It is hard to come up with a plausible account of why the US economy is still growing relatively slowly and there has been a huge fall in labour participation, without invoking problems from the financial system. Not everyone agrees with this; there are some economists to whom the classical dichotomy is almost literally an article of faith.
V is not constant
Thirdly, the seemingly innocuous assumption that V, the velocity of circulation, is constant, is also false. What does V actually mean? It captures the extent to which people use money (whatever definition we pick) in transacting economic exchange. But this varies both as a result of consumer behaviour (short term changes in keeping money under the bed or in the bank, waiting till the end of the month to make payments etc) and over the longer term owing to changes in financial technology. As my old friend Roy Cromb (an economist at a macro hedge fund, where they think a bit more deeply about these things – at least I hope they do) once pointed out in a Cambridge economics supervision, if everyone moved towards using credit cards for most of their spending, the velocity of circulation would increase. Why? Well each month you would pay a single credit card bill, for which you’d transfer a single amount out of your bank account. You could keep most of your money in a savings account. Very little physical money would be taken out of ATMs because you’re paying using plastic. So the volume of money in circulation would fall (relative to before the use of credit cards) while the volume of nominal output was unchanged. V, being the ratio of the unchanged flow to the lower stock, would therefore increase.
If this sounds too fanciful, we can just look at the actual behaviour of V from the Federal Reserve’s FRED database.
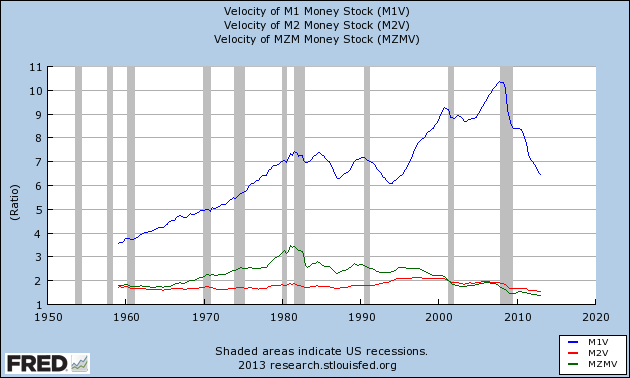
We see here V for each of three different definitions of money supply, M1, M2 and MZM. V not only varies for each one, but the relationship between the different Vs is all over the place. V is very far from constant or stable. Some economists try to model and predict the behaviour of V but there is, to my knowledge, no convincing statistical explanation of V.
So, to return to our simple equation (1): MV=PT.
M is hard to define and measure.
V is unpredictable
It is hard to believe that T is unaffected by the amount of M, at least during economic downturns.
So our nice, simple relationship between M and P has vanished. Most economists would broadly agree that in the long run there is some relationship between the growth of money supply and inflation. But this relationship can break down and even reverse during periods of unusual economic disruption like the rich countries have experienced in the last five years.
Monetarism RIP
The quantity theory lay at the base of the monetarist policy recommendations of Milton Friedman, whose ideas were implemented (imperfectly he argued) by the Bank of England in the 1980s. It is still often claimed that Friedman’s statistical work on money and inflation was sound, even if the central banks were unable to operationalise it. But that is not the case. Just recently, the Oxford econometrician (and one of my lecturers) Prof. David Hendry wrote to the Financial Times to point out that Friedman’s work did not stand up to thorough econometric testing. Hendry was one of those who destroyed the evidence-based case for monetarism, though that is not to say that the volume of money in the economy doesn’t matter.
So this is why the inflation-predicting hedgies have, so far, been wrong. It takes a more complex economic model to explain what has been happening and why the Fed and Bank of England have been able to hugely increase their balance sheets and the amount of money on some definitions, with no increase in inflation. Nobody, least of all the central banks, suggests that these policy experiments carry no risks. But if you have been shorting government bonds for the last four year on the back of a conviction that more money must mean more inflation, you have lost a lot of money – real money that is.
*
Note 1. In the analysis of Prof Michael Woodford, one of the world’s leading monetary economists, the various measures available to central banks when interest rates hit the zero lower bound are known as “unconventional monetary policy”. There are two types: i) forward guidance (providing detailed and credible statements of future intent); and ii) changing the size and structure of the central bank’s balance sheet. “Quantitative easing”, a term invented by the Bank of Japan in the 1990s, refers to one aspect of the latter, but is a useful shorthand for all the central banks’ attempts to stimulate economic activity when interest rates cannot be cut further.


Dan
“Macro-tourists” did not fully understand the transmission of monetary policy and bet wrong- long gold and short treasuries.
Thank you for this eloquent explanation.
Miriam Gordon
I now realize my mistake, alongside the hedge fund managers, about assuming a link between monetary supply and inflation. But do you think there is perhaps an element of truth. Quantitative Easing allows banks to make more loans, which could be blamed for asset bubbles. If a market is experiencing high growth, and loans are readily available, is the upwards spiral of price (many times larger than underlying value of the asset) and investment not inevitable?
Simon Taylor
QE doesn’t lead to more bank loans, the volume of which is under the banks’ own control. In the weak Eurozone economy and still to some extent in the UK, more bank lending would in any case be desirable. But QE is not a way of achieving it.